Answer
Calculus, 10th Edition (Anton) answers to Chapter 2 - The Derivative - 2.2 The Derivative Function - Exercises Set 2.2 - Page 132 40 including work step by step written by community members like you. Textbook Authors: Anton, Howard, ISBN-10: 0-47064-772-8, ISBN-13: 978-0-47064-772-1, Publisher: Wiley. 2.4 Connections f, f' and Trig Notes 2.4 Key. Powered by Create your own unique website with customizable templates. 4.4 Areas, Integrals and Antiderivatives Contemporary Calculus 3 To evaluate a definite integral ⌡ ⌠ a b f(t) dt, we can find any antiderivative F of f and evaluate F(b) – F(a). This result is a special case of Part 2 of the Fundamental Theorem of Calculus, and it will be used hundreds of times in the next several chapters. 4.3 Connecting f ' and f ' with the graph of f Calculus Using the results from the last few statements leads us to the following definition. Definition of Concavity Let y = f (x) be a differentiable function on an interval I.
Work Step by Step
25=2!2!2!2!2=32 26=2!2!2!2!2!2=64 c. They are half as large each time. Divided by 2, or multiplied by ½ is also acceptable. 20=2!1 2 =1,!2'1=1!1 2 =1 2,2'2=1 2!1 2 =1 2,!2'3=1 4!1 2 =1 8,!2'4=1 8!1 2 =1 16 2'n=1 2n'1!1 21 =1 2n'1+1 =1 2n 1-9. 2!4'22=2!2!!!Check:!1 16 '4=1 4 b. 2!1'2!2=2!3Check:!1 2 '1 4 =1 8 c.
Section 5-4 : Line Integrals of Vector Fields
In the previous two sections we looked at line integrals of functions. In this section we are going to evaluate line integrals of vector fields. We’ll start with the vector field,
[vec Fleft( {x,y,z} right) = Pleft( {x,y,z} right)vec i + Qleft( {x,y,z} right)vec j + Rleft( {x,y,z} right)vec k]and the three-dimensional, smooth curve given by
[vec rleft( t right) = xleft( t right)vec i + yleft( t right)vec j + zleft( t right)vec khspace{0.25in}hspace{0.25in}a le t le b]The line integral of (vec F) along (C) is
[intlimits_{C}{{vec Fcenterdot d,vec r}} = int_{{,a}}^{{,b}}{{vec Fleft( {vec rleft( t right)} right)centerdot vec r'left( t right),dt}}]Note the notation in the integral on the left side. That really is a dot product of the vector field and the differential really is a vector. Also, (vec Fleft( {vec rleft( t right)} right)) is a shorthand for,
[vec Fleft( {vec rleft( t right)} right) = vec Fleft( {xleft( t right),yleft( t right),zleft( t right)} right)]We can also write line integrals of vector fields as a line integral with respect to arc length as follows,
[intlimits_{C}{{vec Fcenterdot d,vec r}} = intlimits_{C}{{vec Fcenterdot vec T,ds}}]where (vec Tleft( t right)) is the unit tangent vector and is given by,
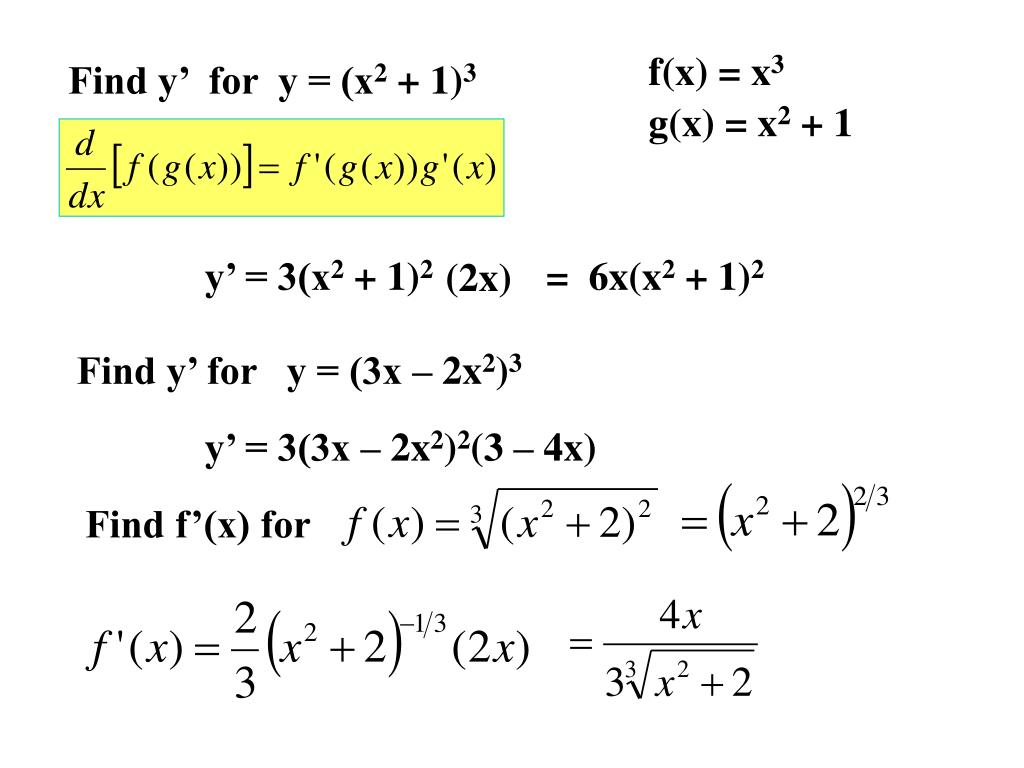 [vec Tleft( t right) = frac{{vec r'left( t right)}}{{left| {vec r'left( t right)} right|}}]
[vec Tleft( t right) = frac{{vec r'left( t right)}}{{left| {vec r'left( t right)} right|}}] If we use our knowledge on how to compute line integrals with respect to arc length we can see that this second form is equivalent to the first form given above.
[begin{align*}intlimits_{C}{{vec Fcenterdot d,vec r}} & = intlimits_{C}{{vec Fcenterdot vec T,ds}} & = int_{{,a}}^{{,b}}{{vec Fleft( {vec rleft( t right)} right)centerdot frac{{vec r'left( t right)}}{{left| {vec r'left( t right)} right|}},left| {vec r'left( t right)} right|,dt}} & = int_{{,a}}^{{,b}}{{vec Fleft( {vec rleft( t right)} right)centerdot vec r'left( t right),dt}}end{align*}]In general, we use the first form to compute these line integral as it is usually much easier to use. Let’s take a look at a couple of examples.
Example 1 Evaluate ( displaystyle intlimits_{C}{{vec Fcenterdot d,vec r}}) where (vec Fleft( {x,y,z} right) = 8{x^2}y,z,vec i + 5z,vec j - 4x,y,vec k) and (C) is the curve given by (vec rleft( t right) = t,vec i + {t^2},vec j + {t^3},vec k), (0 le t le 1). Show Solution
Evaluate ( displaystyle intlimits_{C}{{vec Fcenterdot d,vec r}}) where (vec Fleft( {x,y,z} right) = 8{x^2}y,z,vec i + 5z,vec j - 4x,y,vec k) and (C) is the curve given by (vec rleft( t right) = t,vec i + {t^2},vec j + {t^3},vec k), (0 le t le 1). Show Solution
Okay, we first need the vector field evaluated along the curve.
[vec Fleft( {vec rleft( t right)} right) = 8{t^2}left( {{t^2}} right)left( {{t^3}} right)vec i + 5{t^3},vec j - 4tleft( {{t^2}} right)vec k = 8{t^7},vec i + 5{t^3},vec j - 4{t^3},vec k]Next, we need the derivative of the parameterization.
[vec r'left( t right) = ,vec i + 2t,vec j + 3{t^2},vec k]
Finally, let’s get the dot product taken care of.
[vec Fleft( {vec rleft( t right)} right)centerdot vec r'left( t right) = 8{t^7} + 10{t^4} - 12{t^5}]The line integral is then,
[begin{align*}intlimits_{C}{{vec Fcenterdot d,vec r}} & = int_{{,0}}^{{,1}}{{8{t^7} + 10{t^4} - 12{t^5},dt}} & = left. {left( {{t^8} + 2{t^5} - 2{t^6}} right)} right|_0^1 & = 1end{align*}] Example 2 Evaluate ( displaystyle intlimits_{C}{{vec Fcenterdot d,vec r}}) where (vec Fleft( {x,y,z} right) = x,z,vec i - y,z,vec k) and (C) is the line segment from (left( { - 1,2,0} right)) to (left( {3,0,1} right)). Show SolutionWe’ll first need the parameterization of the line segment. We saw how to get the parameterization of line segments in the first section on line integrals. We’ve been using the two dimensional version of this over the last couple of sections. Here is the parameterization for the line.
[begin{align*}vec rleft( t right) & = left( {1 - t} right)leftlangle { - 1,2,0} rightrangle + tleftlangle {3,0,1} rightrangle & = leftlangle {4t - 1,2 - 2t,t} rightrangle ,hspace{1.0in} 0 le t le 1end{align*}]So, let’s get the vector field evaluated along the curve.
[begin{align*}vec Fleft( {vec rleft( t right)} right) & = left( {4t - 1} right)left( t right),vec i - left( {2 - 2t} right)left( t right),vec k & = left( {4{t^2} - t} right)vec i - left( {2t - 2{t^2}} right)vec kend{align*}]Now we need the derivative of the parameterization.
[vec r'left( t right) = leftlangle {4, - 2,1} rightrangle ]The dot product is then,
[vec Fleft( {vec rleft( t right)} right)centerdot vec r'left( t right) = 4left( {4{t^2} - t} right) - left( {2t - 2{t^2}} right) = 18{t^2} - 6t]The line integral becomes,
[begin{align*}intlimits_{C}{{vec Fcenterdot d,vec r}}& = int_{{,0}}^{{,1}}{{18{t^2} - 6t,dt}} & = left. {left( {6{t^3} - 3{t^2}} right)} right|_0^1 & = 3end{align*}]Let’s close this section out by doing one of these in general to get a nice relationship between line integrals of vector fields and line integrals with respect to (x), (y), and (z).
Given the vector field (vec Fleft( {x,y,z} right) = P,vec i + Q,vec j + R,vec k) and the curve (C) parameterized by (vec rleft( t right) = xleft( t right)vec i + yleft( t right)vec j + zleft( t right)vec k), (a le t le b) the line integral is,
[begin{align*}intlimits_{C}{{vec Fcenterdot d,vec r}} & = int_{{,a}}^{{,b}}{{left( {P,vec i + Q,vec j + R,vec k} right)centerdot left( {x',vec i + y',vec j + z',vec k} right),dt}} & = int_{{,a}}^{{,b}}{{Px' + Qy' + Rz',dt}} & = int_{{,a}}^{{,b}}{{Px',dt}} + int_{{,a}}^{{,b}}{{Qy',dt}} + int_{{,a}}^{{,b}}{{Rz',dt}} & = intlimits_{C}{{P,dx}} + intlimits_{C}{{Q,dy}} + intlimits_{C}{{R,dz}} & = intlimits_{C}{{P,dx}} + Q,dy + R,dzend{align*}]So, we see that,
Note that this gives us another method for evaluating line integrals of vector fields.
This also allows us to say the following about reversing the direction of the path with line integrals of vector fields.
Fact
2.4 Connections F F' And Trigap Calculus Solutions
This should make some sense given that we know that this is true for line integrals with respect to (x), (y), and/or (z) and that line integrals of vector fields can be defined in terms of line integrals with respect to (x), (y), and (z).
